@mrdk @unnick I'm kind of surprised and not surprised about how the tetrahedron turned out.
#MathArt #geometry #animation #loop #geogebra #tetrahedron #3d
Recent searches
Search options
#geometry
@mrdk @unnick And here’s the rhombic triacontahedron for the dodecahedron/icosahedron (again without scaling the bars to have constant length).
#MathArt #geometry #animation #loop #geogebra #dodecahedron #icosahedron #3d
@mrdk @unnick This version shows how the cube/octahedron works using a rhombic dodecahedron (without scaling the bars to have constant length).
#MathArt #geometry #animation #loop #geogebra #cube #octahedron #3d
@mrdk @unnick
I'm not sure that these are related to the Jitterbug transformation.
This is my recreation of unnick's original cube/octahedron loop. I used the rhombic dodecahedron and rhombic triacontahedron for this and the previous loop. They remind me of tensegrity structures.
BTW, I made a couple of origami versions of the Jitterbug transformation many years ago. This one https://foldworks.net/wp-content/uploads/2018/06/jitterbug.pdf works better than the first version https://britishorigami.org/academic/davidpetty/origamiemporium/lam_jitterbug.htm
I couldn’t resist making this in Geogebra: morphing between a regular icosahedron and a regular dodecahedron.
h/t @unnick https://mathstodon.xyz/@unnick@booping.synth.download/114350750053349050
Floor tiles, #Saigon Central Post Office, Ho Chi Minh City, #Vietnam https://en.m.wikipedia.org/wiki/Saigon_Central_Post_Office
#TilingTuesday #geometry #tiling #MathArt #photography #pattern #architecture
Denver, CO (2022)
#photography #streetphotography #geometry #graphicdesign #layout #abstract
#Crows very good at #geometry, they can not only distinguish the from stars, but choose between distinctive quadrilaterals
https://www.earth.com/news/crows-can-recognize-geometric-patterns-in-shapes/

What a fascinating book about hybercubes!
**Crows Can Do Geometry!**
A new study reveals that carrion crows can recognize geometric patterns like right angles and symmetry—skills once thought unique to humans. These clever birds are reshaping our understanding of animal intelligence.
#GoodNews #AnimalIntelligence #Crows #Geometry #CognitiveScience
https://www.npr.org/2025/04/12/nx-s1-5359438/a-crows-math-skills-include-geometry
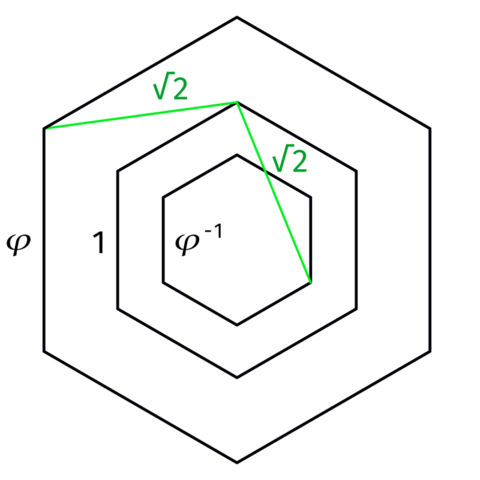
I have found an interesting geometric fact: suppose you have a hexagon of side 1 and duplicate and enlarge it by the golden ratio 𝜑; the distance from one vertex of the unit hexagon to a vertex of the bigger hexagon 60° apart is √2. Furthermore, if another hexagon reduced by 𝜑 is drawn inside, the distance from one vertex of the unit hexagon to a vertex of the smaller hexagon 120° apart is also √2 [first figure].
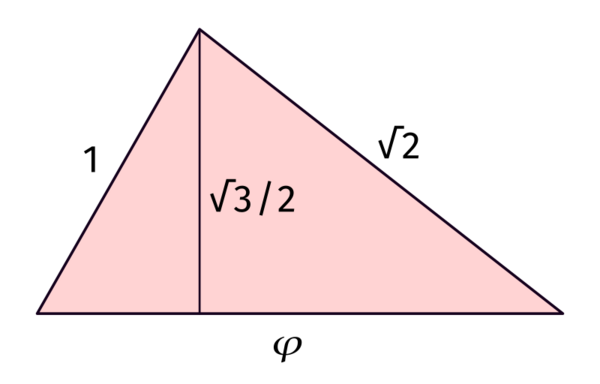
This boils down to the fact that a triangle of sides 1, √2, and 𝜑 has an angle of 60° opposite to side √2. That triangle is very remarkable as it contains the three more relevant algebraic geometric constants: √2, √3/2 (altitude to the bigger side) and 𝜑 [second figure]. Of course this can be also used to construct 𝜑 from a square and a triangle (I bet this is known). In the follow-up some artistic designs exploiting those facts.
#geometry #Mathematics #triangle #GoldenRatio
New blog post! "Go For Geometry! Episode 6: Quadrilaterals"
Using technology (all platforms) to make sense of this family of shapes.
#MTBoS #iTeachMath #T3Learns #Geometry
#ClassroomMath #MathEd #MathsEdChat
https://karendcampe.wordpress.com/2025/04/17/go-for-geometry-6/
Here's a stupid #geometry / #astronomy question. Planetary orbits are ellipses (under the obvious simplifying assumptions) which are conic sections. So what's the cone? There are infinitely many cones that fit of course. But is there one that best explains? To put it another way, is there a neat geometric argument that an orbit should be an ellipse, that doesn't require too much physics? #mathematics
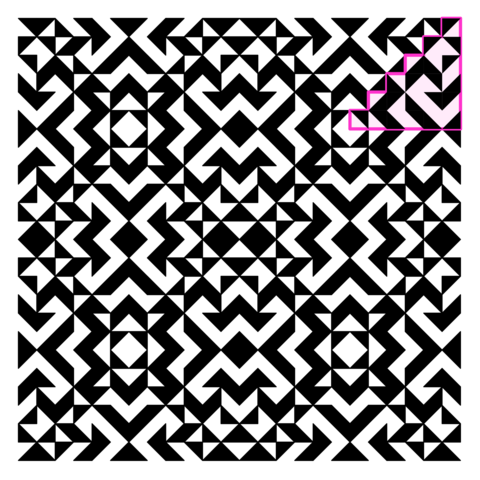
A "big" one :
#geogebra : www.geogebra.org/m/tzmjrbqz
#truchet #mathart #geometry #symetries #reflexion